Compound inequalities

A compound inequality consists of two or more inequalities. There are two types of compound inequalities: compound inequalities joined by the word "and" and compound inequalities joined by the word "or."

Example

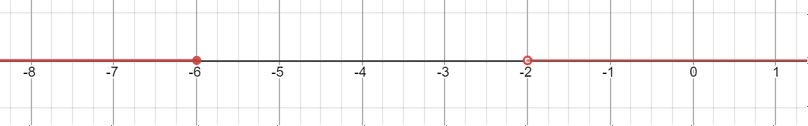
-6 < x ≤ 2

This can also be written as the following, and is represented by the number line below:

x > -6 and x ≤ 2

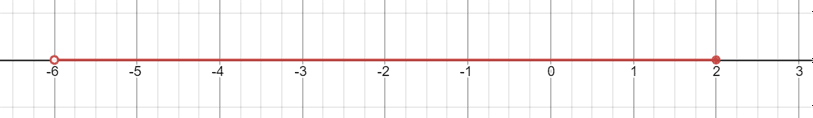
x < -4 ; x ≥ -1

This can also be written as the following, and is represented by the number line below:

x < -4 or x ≥ -1

Compound inequalities joined by "and" are also called the "intersection" of two inequalities. Compound inequalities joined by "or" are also called the "union" of two inequalities.

To solve compound inequalities joined by the word "and," apply the rules for inequalities to all sides of the compound inequality. If you multiple or divide the compound inequality by a negative number, you will need to flip all the inequality signs.

Example

3 ≤ -6x + 9 < 6

-6 ≤ -6x < -3

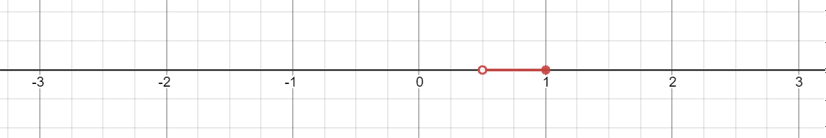
1 ≥ x > ½

½ < x ≤ 1

To solve compound inequalities joined by the word "or," solve each inequality separately. Treat each inequality as its own singular inequality, determine the result of the individual inequalities, then combine the results.

Example

-4x + 5 < 13	or	-2x - 8 ≥ 4
-4x < 8		-2x ≥ 12
x ≤ -6		x > -2