Rectangle

A rectangle is a quadrilateral with four right angles. The following are two examples.

Rectangles are one of the most common shapes you will see in daily life. Many objects around us are rectangular in shape, such as a book, a phone, a door, a card, and many more.

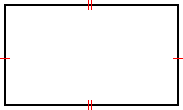
Sides of a rectangle

The opposite sides of a rectangle are congruent and parallel to each other. The longer sides of a rectangle are typically referred to as its length while the shorter sides are referred to as its width.

Angles of a rectangle

A rectangle contains four interior right angles. Angles A, B, C and D equal 90° in the rectangle shown below.

Diagonals of a rectangle

There are two diagonals in a rectangle. The two diagonals (AC and BD in the rectangle below) are congruent and bisect each other. Each diagonal divides the rectangle into two congruent right triangles.

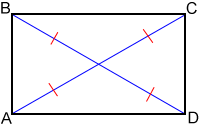
Diagonal BD divides rectangle ABCD into triangles ABD and CDB. AB≅CD and AD≅BC since opposite sides of a rectangle are congruent. ∠A≅∠C since the interior angles of a rectangle are right angles. Therefore, △ABD≅△CDB by the Side-Angle-Side postulate.

Square

A square has four congruent sides and four right angles, so it is also a rectangle. A rectangle is a square when all of its sides are congruent.

Symmetry in a rectangle

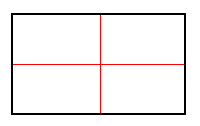
A rectangle has 2 lines of symmetry and a rotational symmetry of order 2, which means that it can be rotated in such a way that it will look the same as the original shape 2 times in 360°.

Lines of symmetry	Rotational symmetry
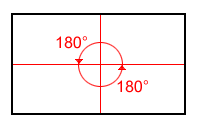2 lines of symmetry	two 180° angles of rotation

Area of a rectangle

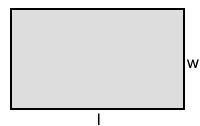
The area of a rectangle is the product of its length and width.

A = l·w

Where l is the length and w is the width of the rectangle. Some textbooks might write the dimensions of a rectangle as base and height instead of length and width.