Dimensions

In mathematics, dimension has two meanings. Dimension is used as a description of the size of an object. For example, the length and width describe the dimension or size of a rectangle. The length, width, and height describe the dimensions, or size, of a rectangular prism.

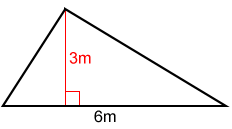
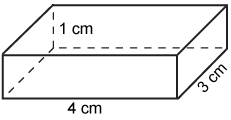
Below are two figures along with their stated dimensions.

The base and height of the triangle above are 6 m and 3 m respectively.		The length, width, and height of the above rectangular prism are 4 cm, 3 cm, and 1 cm respectively.

The dimension of an object is also defined as the minimum number of coordinates needed to specify any point within a mathematical space, or the number of degrees of freedom of a point that moves on the object. A line, for example, has one dimension because a point can only move along the line. We live in what we know to be a 3D world; therefore, most studies in mathematics are limited to 0D, 1D, 2D, and 3D space, as described below.

Zero-dimensional space

A point describes a position in space but has no size or shape, so it has no, or zero dimensions.

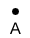
Although a point technically has no size or shape, we use a dot, as shown above, to represent a point.

One-dimensional space

A line or any of its parts (line segment or ray) is one dimensional (1D). In one-dimensional space, any point can be described with one number, as shown in the figure below. Any point on the line can only move along the line. An object in a one-dimensional space, such as a line segment, can be described using just one dimension, length.

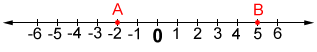
The number line above is 1D. A point can only move left or right along the line. The position of point A can be described with just one coordinate number, -2. The size of AB can be described by its length, 7.

Two-dimensional space

A 2D object has length and width, but no depth. A plane has two-dimensions, length and width and forms a flat surface (such as a piece of notebook paper) extending in both directions infinitely, as shown below.

In a two-dimensional space, any point can be described with two numbers. Any point on a two-dimensional space has two degrees of freedom; a point can move from one position to any position in the space through a combination of moving horizontally and vertically.

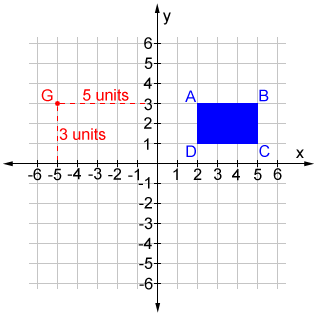
Point G above is graphed in the 2D coordinate plane by moving 5 units left of the origin along the x-axis and 3 units up along the y-axis. The position of G can be described with two numbers (-5, 3). The size of an object, such as the blue rectangle ABCD above, can be described using width and length.

Three-dimensional space

A 3D object has length, width, and depth. The rectangular prism shown below has length, width, and height. Each dimension is measured as a length that is perpendicular to any other dimension. In this case, the width is the depth of the prism.

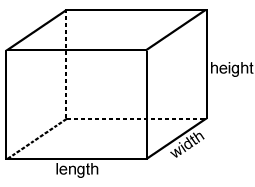
In a three-dimensional space, any point can be described with three numbers. Any point on a three-dimensional space has three degrees of freedom.

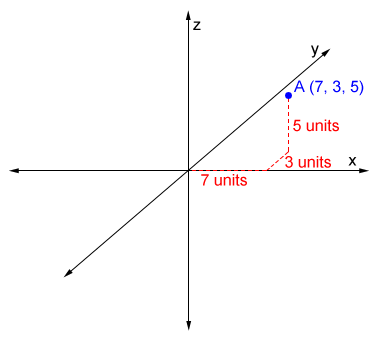
Point A is graphed in the 3D coordinate plane by moving 7 units in the positive direction along the x-axis, 3 units in the positive direction along the y-axis, and 5 units up along the z-axis. The position of A can be described with three numbers (7, 3, 5).