Congruent
In Geometry, two or more figures or objects are congruent if they have the same size and shape, usually referring to line segments, shapes/figures, and angles. For example, line segments with the same length are congruent, and angles with the same measure are congruent. Below are three sets of congruent geometric figures.
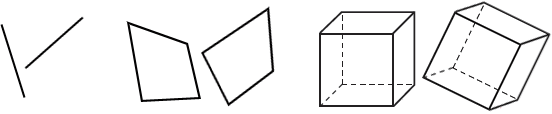
The mathematical symbol "≅" is used to show congruence.
Congruent line segments
Two line segments are congruent if they have the same length.
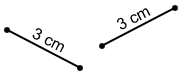
Line segment AB and CD above are congruent since each has a length of 3 cm; AB≅CD. The line segments do not need to be parallel to be congruent.
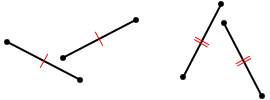
Short line segments, called tick marks, are commonly used to denote the congruence of line segments, as shown in the figure below.
Congruent angles
Two-angles are congruent if they have the same angle measure.

Congruent angles can also be denoted by placing an equal number of arcs between the rays that form the congruent angles. Tick marks (like those used with congruent line segments) can also be used along with arcs, as shown in the figure below.
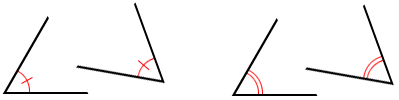
When two figures are congruent, their corresponding parts are equal in value including side, edge, angles, faces, area, volume, and more. There are many types of figures; the following are just 2 examples.
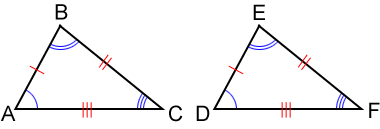
For the two congruent triangles above, the following angles and sides are congruent: ∠A≅∠D, ∠B≅∠E, ∠C≅∠F; AB≅DE, BC≅EF, AC≅DF.
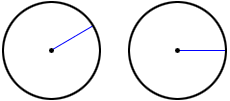
For the two congruent circles above, the radius, area, and circumference are equal in value.
Producing congruent figures
There are three transformations that produce congruent figures. These transformations can be combined.
Translation
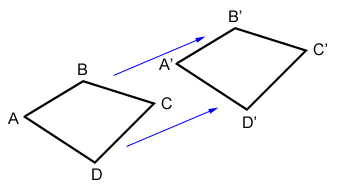
If quadrilateral ABCD is translated diagonally to quadrilateral ABCD' then, quadrilateral ABCD ≅ quadrilateral ABCD' since both quadrilaterals have the same size and shape.
Rotation
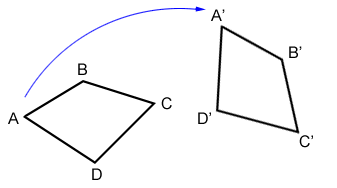
If quadrilateral ABCD is rotated clockwise to quadrilateral ABCD', then, ABCD ≅ ABCD' since both quadrilateral have the same size and shape.
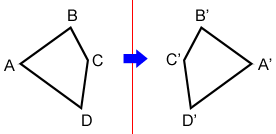
Reflection
If quadrilateral ABCD is reflected across the red line below to quadrilateral ABCD', then, ABCD ≅ ABCD' since both triangles have the same size and shape.
Proving congruence
Two figures are congruent if their corresponding parts are congruent. However, it is usually not necessary to know that every part is equal in order to prove congruence. Proving congruence differs for different shapes. For example:
- Two squares are congruent if their sides are equal, perimeters, or areas are equal.
- Two circles are congruent if their radii, diameters, areas, or their circumferences are equal.
Refer to congruent triangles to see the methods of determining the congruence of triangles.
